PhD defense of Adèle VEILLEUX : ” Extension of the spectral difference method to simplex cells and hybrid grids”
Wednesday 24 March 2021 at 15h00
Phd Thesis Cerfacs, Toulouse (France) - Salle de réunion Administration en visioconférence
Webex link:
https://cerfacs.webex.com/cerfacs/j.phpMTID=md84fc463705118179ca351bdd80ee805
access code: 128 243 1066
password: rXN5DAMGj63
Please switch off your camera and micro during the defense except for the jury’s members
This thesis examines the extension of the Spectral Difference (SD) method on unstructured hybrid grids involving simplex cells (triangles, tetrahedra) and prismatic elements. The Spectral Difference method is part of high-order spectral discontinuous numerical methods. These methods rely on piecewise continuous polynomial approximation to obtain high-order accuracy with a good parallel efficiency. The standard SD scheme is first presented in the one-dimensional case and then for tensor-product elements (quadrangles and hexahedra). The treatment of simplex cells using Raviart-Thomas elements is detailed for triangles (in 2D) and tetrahedra (in 3D), followed by the implementation for prismatic elements.
The linear stability of the Spectral Difference method using Raviart-Thomas elements (SDRT) is studied on triangles and tetrahedra. The SDRT scheme stability is strongly dependent on the interior flux points location. On triangles, the SDRT implementation based on interior flux points located at Williams-Shunn-Jameson quadrature points is found stable up to the fourth-order of accuracy but shown as spatially unstable for higher orders. Nevertheless, it is shown that this implementation can be stabilized for fifth- and sixth-order schemes using suitable temporal integration schemes. This approach being submitted to strict conditions, an optimization of the interior flux points location is conducted to determine spatially stable SDRT formulations for orders higher than four. The optimization process leads to spatially stable schemes up to the sixth-order of accuracy. Finally, the stability analysis on tetrahedra proves that the SDRT scheme based on the interior flux points located at Shunn-Ham quadrature points is stable up to the third-order.
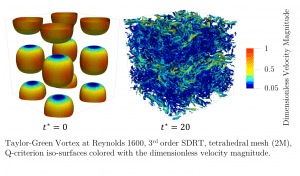
The SD/SDRT numerical method is validated on several academic cases for first and second-order Partial Differential Equations (linear advection equation, Euler equations, Navier-Stokes equations). Both proposed implementations (based either on Williams-Shunn-Jameson quadrature points or optimization points) are used. Numerical experiments involve grids composed of quadratic triangles, linear tetrahedral elements as well as 2D hybrid meshes.
Keywords : High-order discontinuous method, Spectral Difference, Hybrid grid, Stability analysis, Coupled time-space analysis
Jury:
| Chunlei LIANG | Clarkson University, Postdam-New York, USA | Referee |
| Georg MAY | Von Karman Institute, Sint-Genesius-Rode, Belgium | Referee |
| Hélène BARUCQ | INRIA Toulouse, France | Member |
| Eusebio VALERO | Universidad Politécnica de Madrid, Spain | Member |
| Hugues DENIAU | ONERA Toulouse, France | Invited member |
| Jean-Luc ESTIVALEZES | IMFT Toulouse, France | Invited member |
| Guillaume PUIGT | ONERA Toulouse, France | Advisor |
| Guillaume DAVILLER | CERFACS Toulouse, France | Co Advisor |
No content defined in the sidebar.

